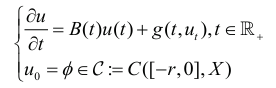INVARIANT STABLE MANIFOLDS FOR PARTIAL FUNCTIONAL DIFFERENTIAL EQUATIONS WITH FINITE DELAY
Abstract
We prove the existence of invarinat stable manifolds for the solutions to the equation
in the case where the evolution family (U(t,s))t≥s≥0 has an exponential dichotomy on a half-line, and the nonlinear forcing term g satisfies the 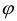
References
J. Hadamard, “Sur l’interation et les solutions asymptotiques des equations differentielles”. Bull. Soc. Math. France, 1901, 29, pp. 224-228.
O. Perron, “Uber Stabilit at und asymptotiques Verhalten der Integrale von Differential gleichungs systemen”. German Math. Z, 1929, 29, no. 1, pp. 129-160.
N.V. Minh, J. Wu, “Invariant manifolds for partial functional differential equations”. J. Differential Equations, 2004, 198, pp. 381-421.
N.T.T. Hang, T.X. Yen, “Conditions for existence of solutions for partial functional delay differential equations on a half-line”. UTEHY Joural of Science and Technology, 2023, 38, pp. 85-90.
N.T. Huy, “Invariant manifolds of admissible classes for semi-linear evolution equations”. J. Differential Equations, 2009, 246, pp. 1820-1844.
A. Pazy, Semigroup of Linear Operators and Application to Partial Differential Equations, Spinger-Verlag, Berlin, 1983.